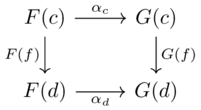
For each object \(c \in \mathcal{C}\), one specifies a morphism \(F(c)\xrightarrow{\alpha_c}G(c)\) in \(\mathcal{D}\) called the c-component of \(\alpha\)
These components must satisfy the naturality condition: for each morphism \(c \xrightarrow{f} d\) in \(\mathcal{C}\) we need \(F(f);\alpha_d=\alpha_c;G(f)\)
AKA this diagram should commute: